Note
Go to the end to download the full example code.
电路#
将布尔电路转换为等效的布尔公式。
在最坏情况下,布尔电路的表达能力可能比等效的公式指数级更高,因为电路可以多次重用子电路,而公式不能多次重用子公式。因此,如果电路较大,通过这种方式从布尔电路创建布尔公式可能是不可行的。
import matplotlib.pyplot as plt
import networkx as nx
def circuit_to_formula(circuit):
# 将电路转换为等效公式。
formula = nx.dag_to_branching(circuit)
# 将每个节点的操作符或变量标签进行转移
# circuit to the formula.
for v in formula:
source = formula.nodes[v]["source"]
formula.nodes[v]["label"] = circuit.nodes[source]["label"]
return formula
def formula_to_string(formula):
def _to_string(formula, root):
# 如果没有子节点,这是一个变量节点。
label = formula.nodes[root]["label"]
if not formula[root]:
return label
# 否则,这是一个运算符。
children = formula[root]
# 如果只有一个子节点,标签必须是NOT运算符。
if len(children) == 1:
child = nx.utils.arbitrary_element(children)
return f"{label}({_to_string(formula, child)})"
# 注意:这里的“左”和“右”有点误导性:实际上
# 节点子节点之间没有顺序。这没关系,因为
# 布尔运算符AND和OR是对称的。这只是意味着
# 操作数的顺序无法预测,因此
# 函数在每个平台上不一定表现相同
# invocation.
left, right = formula[root]
left_subformula = _to_string(formula, left)
right_subformula = _to_string(formula, right)
return f"({left_subformula} {label} {right_subformula})"
root = next(v for v, d in formula.in_degree() if d == 0)
return _to_string(formula, root)
创建一个示例布尔电路。#
这个电路的输出有一个 ∧,下一层有两个 ∨。 第三层有一个变量 x 出现在左边的 ∨ 中,一个 变量 y 出现在左右两个 ∨ 中,以及一个 变量 z 的否定,它作为唯一节点出现 fourth layer.
circuit = nx.DiGraph()
# Layer 0
circuit.add_node(0, label="∧", layer=0)
# Layer 1
circuit.add_node(1, label="∨", layer=1)
circuit.add_node(2, label="∨", layer=1)
circuit.add_edge(0, 1)
circuit.add_edge(0, 2)
# Layer 2
circuit.add_node(3, label="x", layer=2)
circuit.add_node(4, label="y", layer=2)
circuit.add_node(5, label="¬", layer=2)
circuit.add_edge(1, 3)
circuit.add_edge(1, 4)
circuit.add_edge(2, 4)
circuit.add_edge(2, 5)
# Layer 3
circuit.add_node(6, label="z", layer=3)
circuit.add_edge(5, 6)
# 将电路转换为等效公式。
formula = circuit_to_formula(circuit)
print(formula_to_string(formula))
labels = nx.get_node_attributes(circuit, "label")
options = {
"node_size": 600,
"alpha": 0.5,
"node_color": "blue",
"labels": labels,
"font_size": 22,
}
plt.figure(figsize=(8, 8))
pos = nx.multipartite_layout(circuit, subset_key="layer")
nx.draw_networkx(circuit, pos, **options)
plt.title(formula_to_string(formula))
plt.axis("equal")
plt.show()
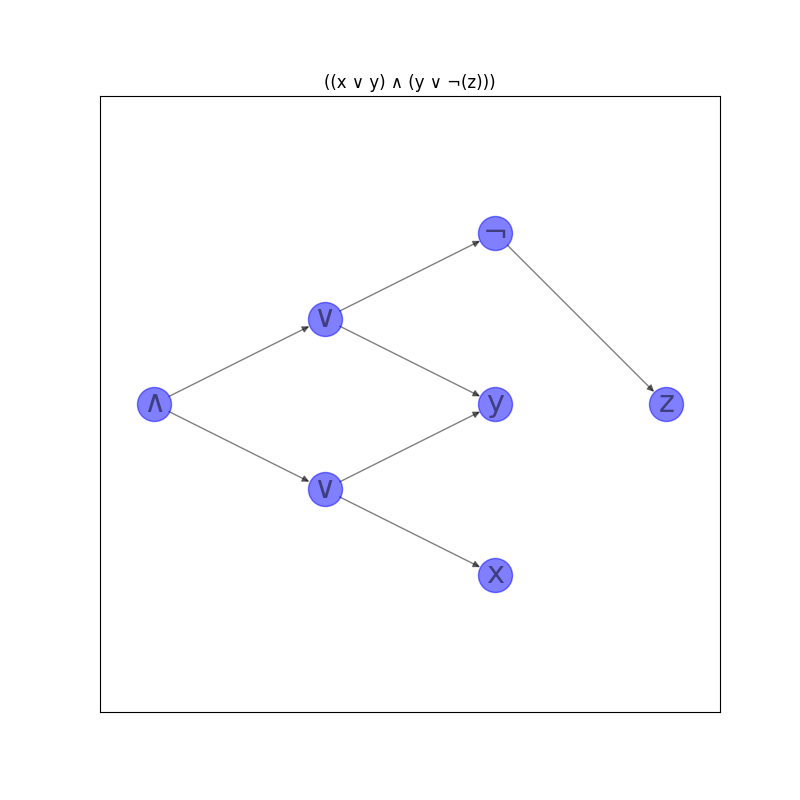
((x ∨ y) ∧ (y ∨ ¬(z)))
Total running time of the script: (0 minutes 0.039 seconds)